Geometry Problem 1579: Unlocking the Secrets of Perpendiculars and Tangents - Calculate the Area of Triangle BCE! A High School and College Challenge

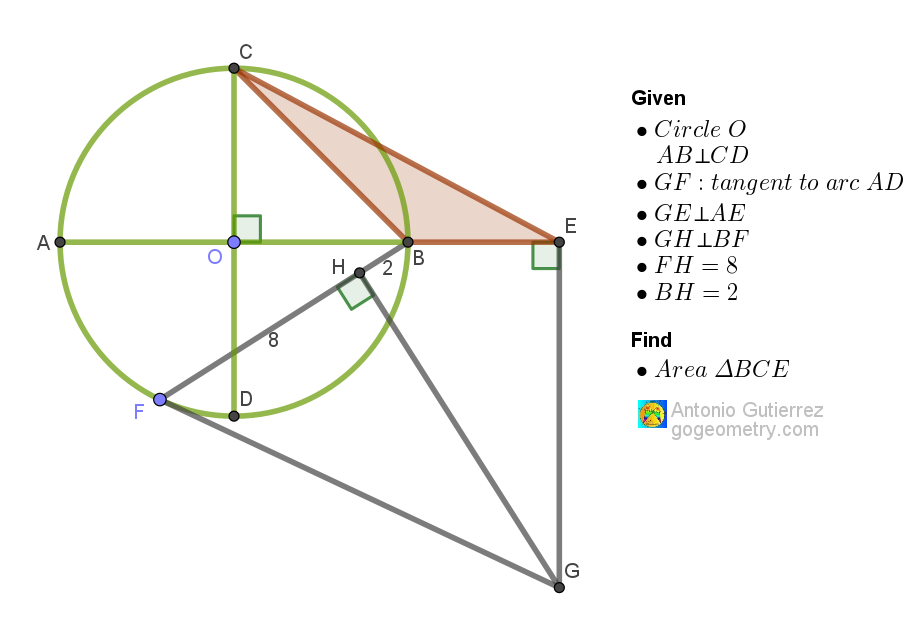
In a circle with center O, diameters AB and CD are perpendicular. The diameter AB is extended to a point E. From a point F on the arc AD, a tangent is drawn to point G, where GE is perpendicular to AE. Line GH is perpendicular to FB. If FH measures 8 units and HB measures 2 units, find the area of triangle BCE.

Uncover and share solutions to this problem.

Hints, Key Definitions and Descriptions

Hints, Key Term	Description
Circle	A set of all points in a plane that are at a given distance from a fixed point, called the center (O in this case).
Diameter	A straight line passing from side to side through the center of a circle. In this problem, AB and CD are diameters.
Perpendicular	Two lines or segments that intersect to form a right angle (90 degrees). In this problem, AB and CD are perpendicular diameters.
Tangent	A line that touches a circle at exactly one point. In this problem, the tangent is drawn from point F to point G.
Radius	A straight line from the center of a circle to any point on its circumference. This term is crucial in solving problems involving circles and tangents.
Right Angle	An angle of 90 degrees. In this problem, GE is perpendicular to AE and GH is perpendicular to FB, forming right angles.
Pythagorean Theorem	A mathematical principle used to find the lengths of sides in a right triangle, expressed as a2 + b2 = c2, where c is the hypotenuse.
Tangent and Radius Theorem	A theorem stating that a tangent to a circle is perpendicular to the radius drawn to the point of tangency. This theorem is used to solve problems involving tangents and radii.
Area of Triangle	The space enclosed by three sides. In this problem, we need to find the area of triangle BCE.

---

Flyer of problem 1579 using iPad Apps