Geometry Problem 1574: Triangle with Three Circles through a Point and the Concyclicity of Six Intersection Points. A High School and College Challenge

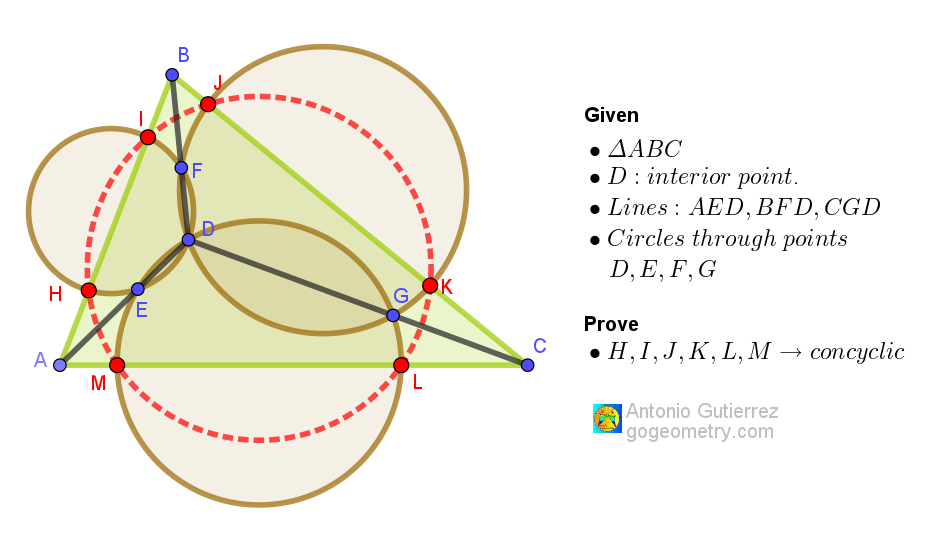
In triangle ABC, let D be an interior point. Points E, F, and G lie on lines AD, BD, and CD, respectively. The circle through E, D, and F intersects AB at H and I; the circle through D, F, and G intersects BC at J and K; the circle through D, E, and G intersects AC at L and M. Prove that H, I, J, K, L, and M are concyclic.

Uncover and share solutions to this problem.

Key Definitions and Descriptions

Term	Description
Interior Point D	A point D located inside triangle ABC.
Points E, F, G	Points lying on lines AD, BD, and CD, respectively.
Circle through E, D, F	A circle passing through points E, D, and F, intersecting AB at points H and I.
Circle through D, F, G	A circle passing through points D, F, and G, intersecting BC at points J and K.
Circle through D, E, G	A circle passing through points D, E, and G, intersecting AC at points L and M.
Concyclic Points H, I, J, K, L, M	Points H, I, J, K, L, and M lie on a common circle, indicating their concyclicity.

---

Flyer of problem 1574 using iPad Apps