Sacred Geometry Art of Problem 1377: Isosceles Triangle, Interior Cevian, Equal Sum of Exradii, Excircle, iPad Apps

Proposition

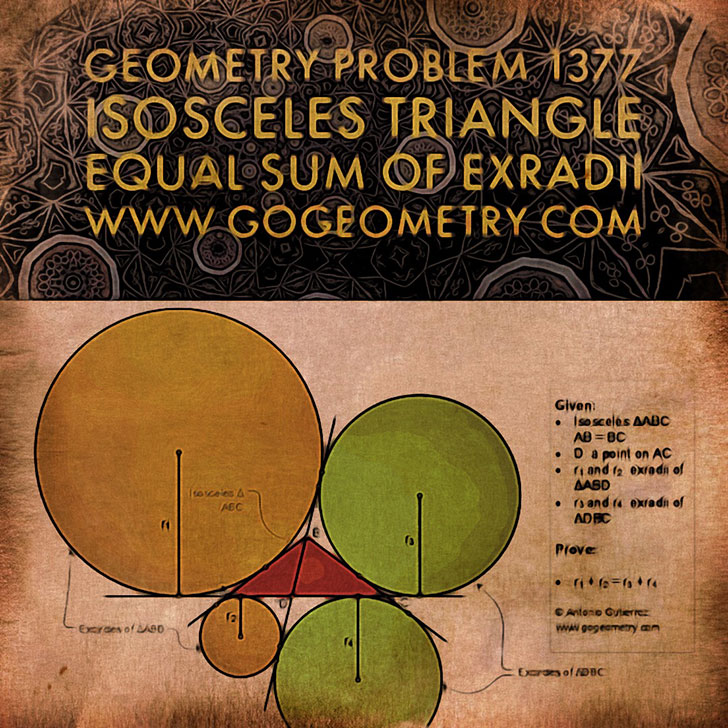
In the figure below, ABC is an isosceles triangle (AB = BC) D is a point on AC, r₁ and r₂ are the exradii of the triangle ABD corresponding to AB and AD, respectively; r₃ and r₄ are the exradii of the triangle DBC corresponding to BC and CD, respectively. Prove that r₁ + r₂ = r₃ + r₄.

BD is called an interior cevian of triangle ABC.

 

See solution below

See also:
Original problem 1377.

Geometric Art using iPad or Mobile Apps

Geometric art is a form of art based on the use and application of geometric figures. A geometric figure is any set or combination of points, lines, surfaces and solids.

A mobile app or mobile application software is a computer program designed to run on smartphones and tablet computers.
.