|
The figure shows circles A (radius r)
and B (radius r) tangent at point C. A common tangent line is
tangent to the circles at points D and E, respectively. Points F
and G are on line segment DE, point M is on arc CD, and point H
is on arc CE. If FGHM is a square of side length x, prove the
following conclusion 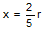 . .
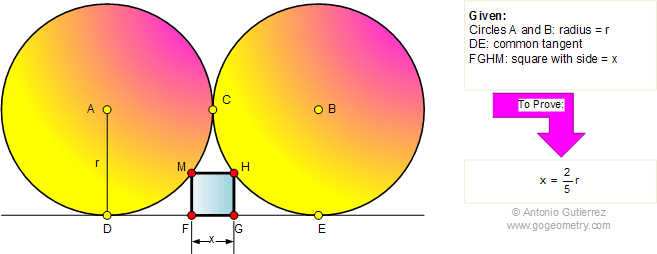
References: Fukagawa Hidetoshi, Tony Rothman, "Sacred
Mathematics: Japanese Temple Geometry" (Princenton
University Press, 2008).
Transform Your Designs with Typography Art in Motion: Create Stunning Visuals with iPad Apps!
Click on the figure below to see the illustration.
|