Geometry Problem 1540: Solving for the Length of Chord in a Circle: Analyzing Intersections and Given Values for Academic Pursuits

Consider a circle with center O and diameter AB. We draw the chord AC. The circle with diameter OC intersects AC at point G and OA at point D. From point A, a line is drawn that intersects CD at point E and the arc BC at point F. Given that AE = 3 and EF = 13, we need to determine the length of AC.

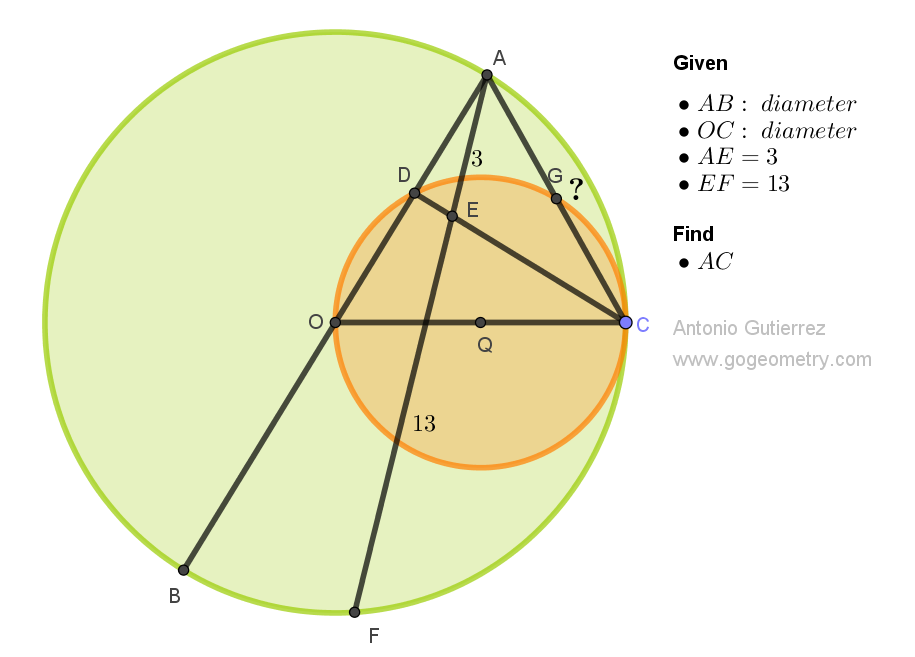
$Geometry Problem 1540: Solving for the Length of Chord in a Circle: Analyzing Intersections and Given Values for Academic Pursuits$

Problem 1540
Intersecting lines,
Circle's secret in their grasp,
Given clues align.
Chord's length, a mystery unveiled,
Geometry's dance unfolds.

View or post a solution

Recent Additions

Geometry Problems
Open Problems
Visual Index
All Problems
Circle
Triangle
Diameter
Tangent Circles
Tangent
Similarity
Metric Relations
View or Post a solution

Discover Even More: See Also...

Problem 1541

Problem 1539

$Geometry Problem 1539 Demystified: Unraveling the Lengths in an Isosceles Triangle with Altitude and Tangent Secrets. Math Class$

Problem 1538

$Geometry Problem 1538: Unlocking the Secrets of Triangular Geometry: Solve for the Area of a Quadrilateral Using External Squares and a Segment Length$

Problem 1537

$Geometry Problem 1537 Challenge: Can You Solve for the Missing Area in a Parallelogram using Midpoints and Intersection Points$

Problem 1536

$Geometry Problem 1536: Discover the Power of Midpoints: Solving for Missing Areas in Quadrilaterals$

Search our site with Google: