|
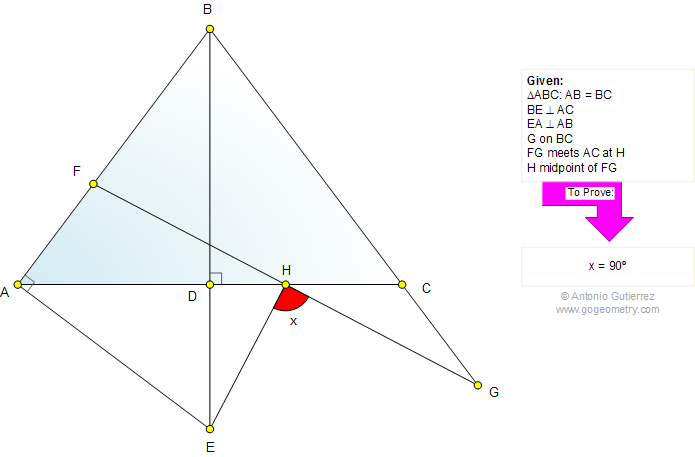
In the diagram below, ABC is an
isosceles triangle (AB = BC). BE is perpendicular to AC and EA
is perpendicular to AB. F is on AB and G is on BC extended. FG
meets AC at H. If H is the midpoint of FG, prove that the
measure of angle EHG is 90 degrees.
|