|
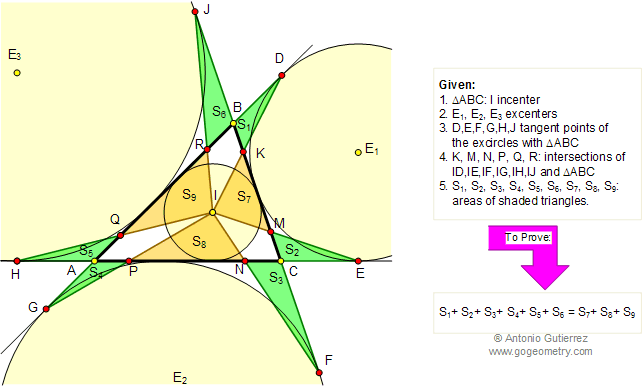
In the figure below, given a triangle
ABC, construct the incenter I and the excircles. Let be D,
E, F, G, H, and J the tangent
points of triangle ABC with its excircles. K, M, N, P, Q, and R
are the intersection points of triangle ABC and ID, IE, IF, IG,
IH, and IJ respectively. If S1, S2, S3,
S4, S5, S6, S7, S8,
and S9, are the areas of the shaded triangles, prove that
S1+S2+S3+S4+S5+S6
= S7+S8+S9.
View or post a solution.
|